The installation of casing is a sequentially repeated process that includes drilling. After each segment of hole has been drilled, casing string of slightly smaller diameter is run in the hole. The annulus between the outer diameter of the pipe and the hole size is then filled with cement, sometimes only in the lower portions of the hole and sometimes all the way from the bottom of the hole back to the surface. Once the cement is placed in the annulus, it takes time for it to set and harden.
During drilling, the annulus is beneficial in that it gives the ability to circulate fluids in the well. Later, however, an annulus can be detrimental if we do not want fluids moving between different parts of the well. As part of designing the well, engineers want to seal the well so that wellbore fluids cannot migrate into the surrounding rocks or between different subsurface zones, and so that only particular fluids from predetermined target zones can migrate into the well (i.e., oil and gas from the reservoir).
The cement in the annulus outside a casing string should keep drilling fluids from flowing up around the outside of the casing, thus protecting the wellbore. Cement is a crucial physical barrier to secure the integrity of the well, preventing ingress of hydrocarbons into the well and loss of well control.
Yet, a Mineral Management Service study of offshore blowouts published in 2007 noted the most common contributing factor for blowouts between 1992 and 2006 was cementing operations. Consequently, cementing operations not only need to be designed according to safety regulations, post-cementing verification tests are required for all wells drilled in the subsurface, which we will discuss in later sections.1US CHEMICAL SAFETY AND HAZARD INVESTIGATION BOARD. (2014, June 5). INVESTIGATION REPORT VOLUME 1 EXPLOSION AND FIRE AT THE MACONDO WELL. US CHEMICAL SAFETY AND HAZARD INVESTIGATION BOARD. REPORT NO. 2010-10-I-OS.
Pumping Cement into the Well
Although the ultimate goal is to fill the annulus around the well casing with cement, cement is actually pumped through the inside of the casing, in the same way that drilling mud passes through the drill string before rising through the annulus as part of mud circulation during drilling.
Naturally, drillers don’t want to end up with the inside of the casing filled with cement, so they carefully calculate how much cement will be needed to fill the annulus outside a given casing string. Prior to running a string of casing, the drill pipe and bit are removed from the hole. With drilling mud still in the hole, the steel pipe that makes up the casing is run to the bottom of the hole. Cement is then pumped into the well through the inside of the casing, displacing the mud ahead of it and returning it to the surface through the annuals. After the specified amount of cement has been pumped into the casing, a ‘wiper plug’ is inserted into the pipe to seal the cement from the following displacement fluid, typically drilling mud. This plug, which works like the end of the plunger in a syringe used for getting shots at the doctor, cleans the cement from the inside of the casing, and in conjunction with the displacement fluid pumped behind it, it pushes the cement out of the bottom of the casing into the annulus. When the plug ‘bumps’ the bottom of the casing string, where there is a special seat installed in an assembly called the shoe, there will be a pressure spike and the drillers will then know to stop pumping. After time is allowed for the cement to start to set, a pressure test will be run to assure the casing is properly sealed. After a successful test, the drill bit will be run back in the hole on the drill string, the wiper plug and shoe will be drilled out of the bottom of the pipe and drilling will continue.
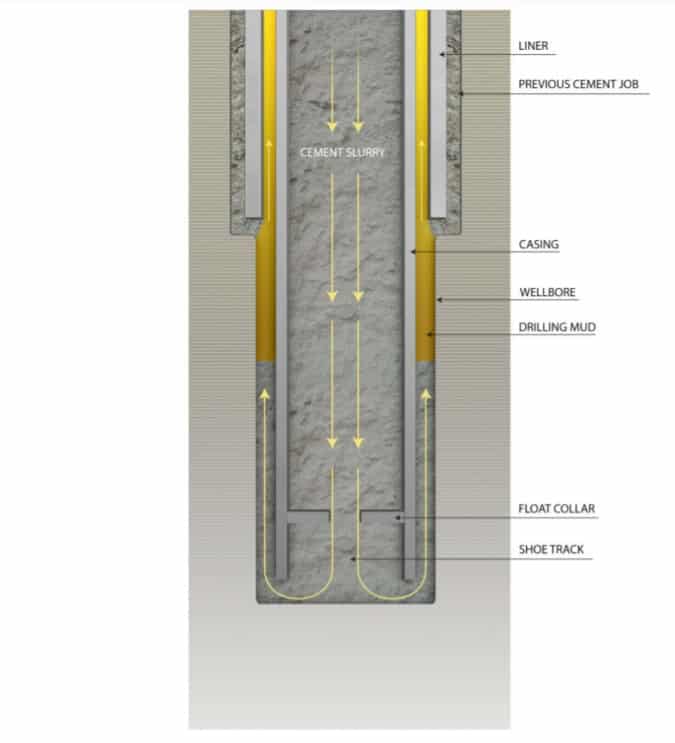
Cementing casing in place involves filling the annulus with cement above any hydrocarbon bearing zones if they are present as well as a small length (30ˈ-90ˈ) in the shoe track.
Calculating the Cement Job
Engineers must calculate the correct amount of cement that is needed to properly fill the annular space using the height of the wellbore and the annular capacity. Let’s take a look at an example of calculating the amount of cement needed for a new well being drilled in the practice problem below.
Practice Problem
Once a segment of the wellbore is drilled, you want to determine how much cement is needed to fill the space between the outside diameter of the casing and the inside diameter of the borehole. Depending on the stage of drilling, part of the borehole may be lined by a previous casing string, while the rest may be lined by bare rock surface as drilled out by the bit, and the two sections may have different inside diameters. For this exercise, we will assume the entire segment to be cased is lined by bare rock.
We can calculate how much cement is needed by determining the annular volume (AV) between the casing string and the hole into which it will be installed. The volume of the annulus can be found by calculating the volume of the cylinder representing the borehole (with diameter DH, indicated as the yellow cylinder in the figure) and subtracting the volume of the cylinder representing the casing string (with diameter DP, indicated as the purple cylinder in the figure) as follows.
\[AV=\left( \left( \pi \frac{DH}{2} \right)^{2} \times height\right) -\left( \left( \pi \frac{DP}{2} \right)^{2} \times height\right) =\frac{\pi }{4} \times \left( DH^{2}-DP^{2}\right) \times height\]
If we were working on a rig, they would report diameter to us in inches and height in ft, so a conversion factor is required to produce the answer of AV in ft3. Modifying the equation to properly compute the answer for the expected units, we can add the conversion factor of 1 ft2 per 144 in2, times the constant in the equation, pi/4, resulting in
\[AV=0.005454\frac{\text{ft}^{2} }{\text{in}^{2} } \times \left( DH^{2}-DP^{2}\right) \times height\]
Assuming we drilled a 17-1/2 inch diameter hole for 16 inch diameter casing over a 5,000 ft hole height, the annular volume of cement required would be
\[AV=0.005454\frac{\text{ft}^{2} }{\text{in}^{2} } \times \left( 17.5\ \text{in}^{2} -16\ \text{in}^{2} \right) \times 5000\ \text{ft} =1370\ \text{ft}^{3}\]
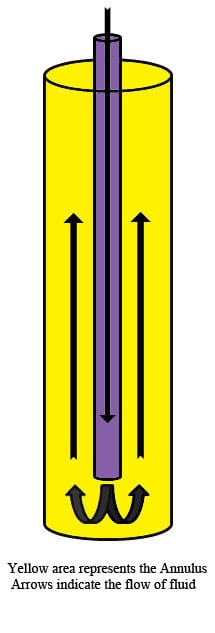
Image Credits
- cement casing in place: U.S. Chemical Safety and Hazard Investigation Board
- annulus diagram: Mudgineer at English Wikipedia, Public domain, via Wikimedia Commons